Astronomical units of measurement are mostly pretty straight-forward:
Astronomical Units refer to the mean Earth-Sun distance (~150 million km or 93 million miles)
Light years are the distance light travels in a year (~9.46 × 10^12 km)
Another astronomical unit of measurement is the parsec. What is a parsec and how is it measured?
A parsec is approximately 3.26 times a light year or 206,265 Astronomical Units (AU).
According to "Cosmic Reference Guide" (Caltech), the word 'parsec' stands for "parallax of one arc second", and is (according to the website):
the length of the long leg of a right triangle, whose short leg is one astronomical unit when the angle between the Sun and the Earth, as seen from an object in space (a star for example), is one arcsecond
Illustrated (from the link above):
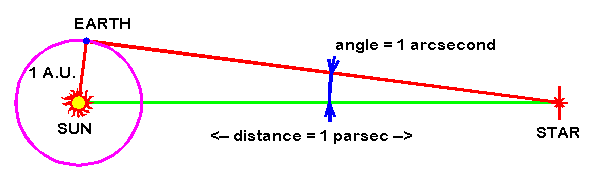
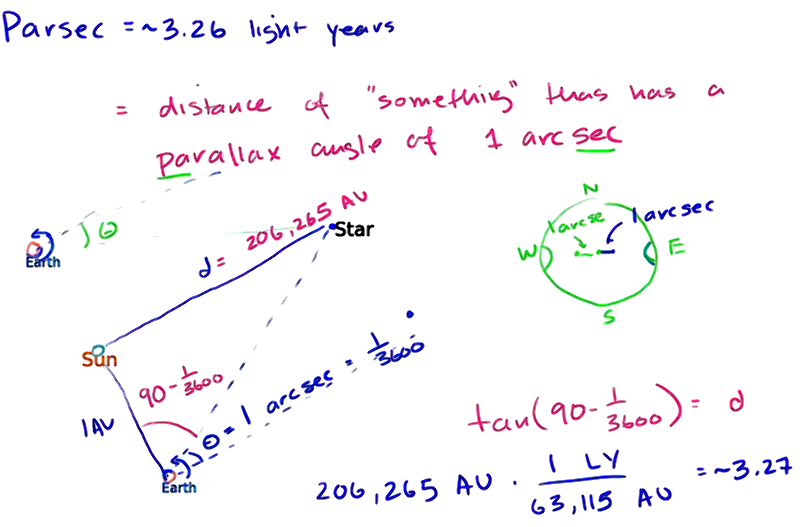
A parsec (abbreviated pc) is a unit of distance used by astronomers, cosmologists, and astrophysicists.
1 parsec is equal to $3.08567758 \times10^{16}$ meters, or $3.26163344$ light years (ly).
A few typical scales to keep in mind:
1) The disks of galaxies like the Milky Way are a few 10's of kpc (that's pronounced kiloparsecs, which are 1000's of parsecs) in size. The dark matter halos surrounding them extend out to almost an order of magnitude further.
2) Galaxy clusters come in at around 1 Mpc (that's pronounced megaparsecs, or millions of parsecs), and are the largest bound objects in the universe. Pictured below is the galaxy cluster Abell 2218.
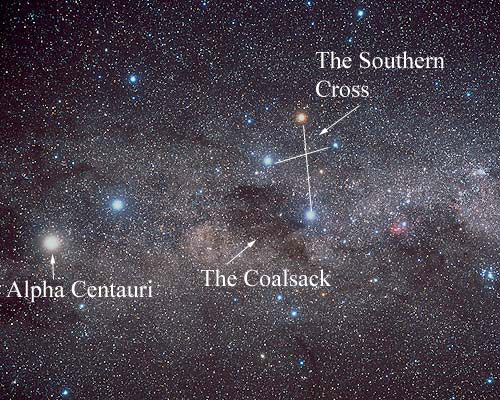
3) The closest star to the planet Earth (not counting the sun, of course) is the star system Alpha Centauri, at a distance of just about 1.3 parsecs. While you may think that this is incredibly close (and it is by cosmological standards), it would nonetheless still take us 4.24 years to travel to it if we traveled at the speed of light.
Parsec or Parallax-arcsecond is defined as the 'distance' of something that has a parallax angle of one arc second. The International Astronomical Union (IAU) define 1 parsec(pc) as:
$$1 \text{pc} = 1/\tan(1'') \ \text{au}$$
$$\Rightarrow 1 \text{pc} = 1/\tan({1\over60}') \ \text{au}$$
$$\Rightarrow 1 \text{pc} = 1/\tan({1\over60\times60}^{\circ}) \ \text{au}$$
$$\Rightarrow 1 \text{pc} = 1/\tan({1\over60\times60} \times {\pi\over180}) \ \text{au}$$
$$\bf \Rightarrow 1 \text{pc} = 206,264.8 \ \text{au}$$
$$\Rightarrow 1 \text{pc} = (2.06265× 10^5) \times (1.496×10^{11}) \ \text{m}$$
$$\bf \Rightarrow 1 \text{pc} = 3.09×10^{16} \ \text{m}$$
$$\Rightarrow1 \text{pc} = 3.09×10^{16} \times {1\over9.46×10^{15}}\text{ly}$$
$$\bf \Rightarrow 1 \text{pc} = 3.26 \ \text{ly}$$
(image source:https://commons.wikimedia.org/wiki/File:Parsec.jpg)
Parsec or Parallax-arcsecond is the distance of something that has a parallax angle of one arc second. Surveying provides an everyday example of parallax. Go outside and look at a distant object. Hold your hand out with one finger raised and close one eye. Line up your finger so it covers the object from view. Then close that eye and open the other without moving your arm. Now, move your finger to block that eye's line of sight. The two positions your finger was at can be used to find the distance from the object. This is what a survey crew does to figure out land plot boundaries. The mathematical technique used in the calculation is trigonometry. A parsec is so large that if you could see that far that you would be looking in to the past. You are looking 3.26 years in the past because it takes 3.26 years for the light to come from a parsec away. I recommend watching the Khan Academy video Parsec definition which I found helpful.
