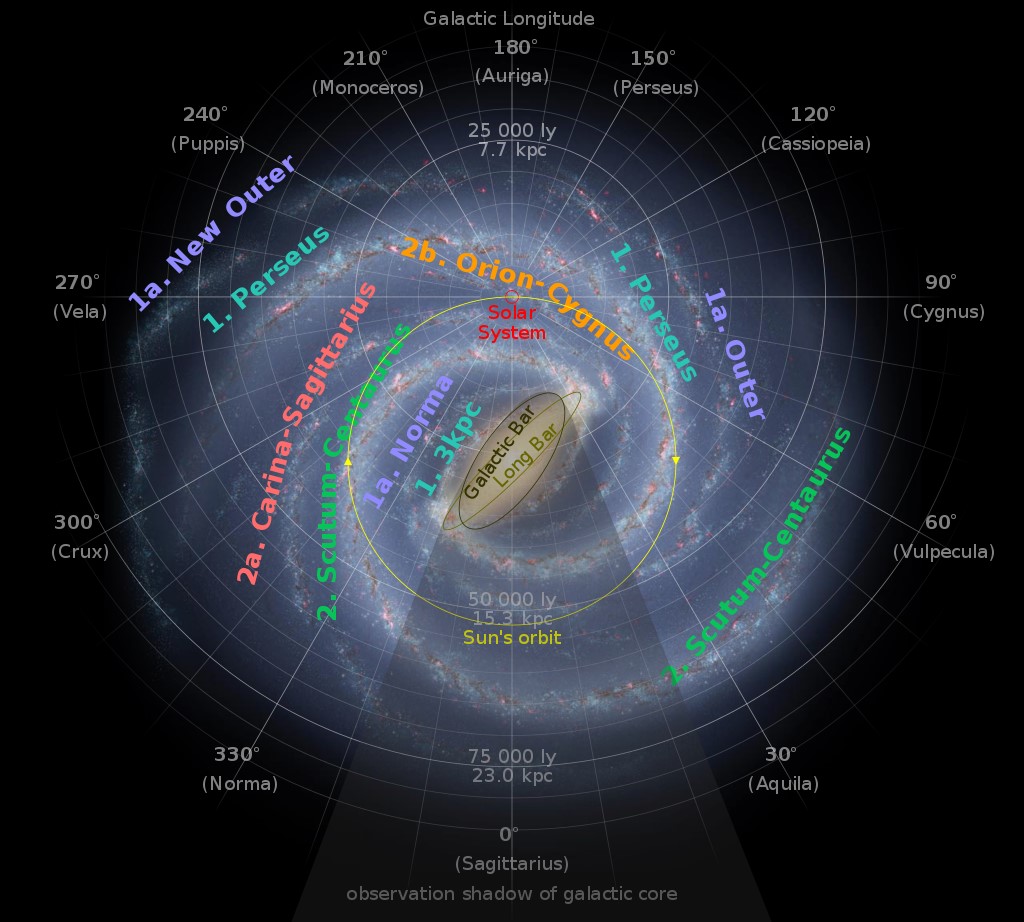
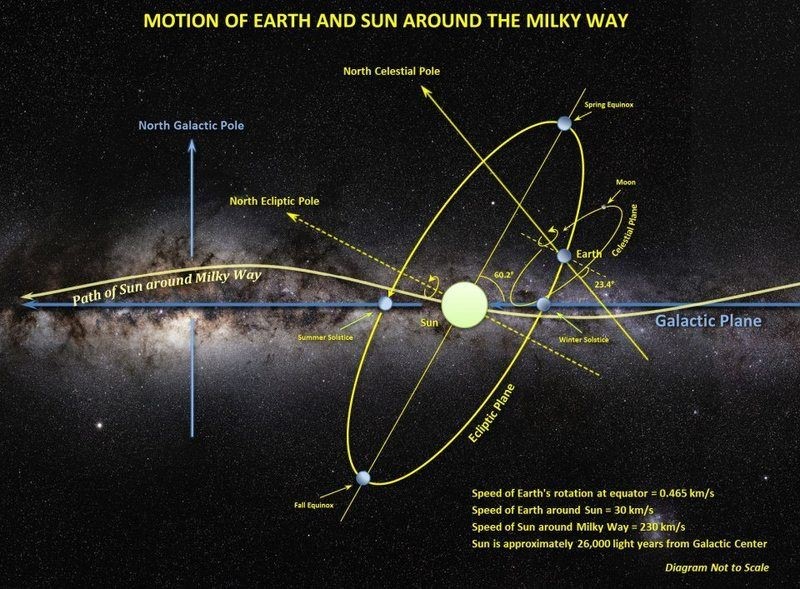
In which direction does the ecliptic plane make an angle of 60 degrees with galactic plane?

Question

In which direction does the ecliptic plane make an angle of 60 degrees with galactic plane? Is it toward the galactic center? (It can have any 360 degree orientation.)

Answer 1

The line defining where the ecliptic plane and the Galactic plane coincide lies (approximately) between the points defined by right ascensions and declinations of 6h +23 and 18h -23.

This can be seen below - the plot is in celestial coordinates with the ecliptic plane marked (thicker solid line) and the Galactic plane clearly visible in the background map of dust extinction.

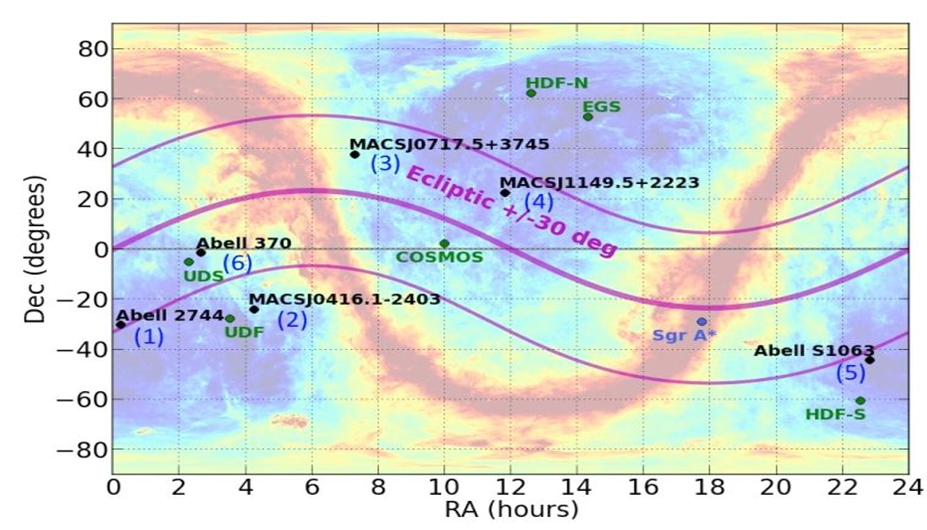
The second of these coordinates is close to, but not coincident, with the Galactic centre (marked Sgr A*). So the relative tilt is sort of at right angles to the Galactic centre at present.

Of course this will change over tens of millions of years as the solar system orbits the Galaxy.

Edit: Another way of specifying the direction is just to ask where the ecliptic north pole is. It is at RA=18h, Dec=66d 34m, in the constellation of Draco.

A further way of thinking about it is to consider at which points in the sky do the ecliptic and Galactic planes have their maximum angular separation. These are 90 degrees away from the two points I defined above and are almost on the celestial equator at about 0h and 12h.

Answer 2

I think that since north ecliptic is l=97 degrees (Lacerta), b=+30 degrees, from earth with 23,5°axis tilt away from center galaxy (celestial sphere is pointing just "only" 3° lower(than ecliptic) toward galaxy disk, b = 27°) it will appear to be Cassiopeia 120°. For stargazing it has more sense consider Celestial sphere be pointing to Cassiopeia (or 27° above (toward galactic north pole) Cassiopeia).

I ve add same pic in bellow as author did, but with stars, which can anyone observe and so can be more familiar with (in appendix it is explained). Milky way is faint there, but you can compare it with the second bellow pic., that shows the constellations in galactic plane surrounding our solar system. Third pic bellow shows Earth position in equinoxes and solstices on its orbit around the sun.

If you are in mid-EU latitude (50°), you may use something like Stellarium and check positions of these planes in solstices and equinoxes (as I did) and it may help you to get feeling for their orbits. Some pictures bellow also may help you to visualize it. If you are on further away lat., you will see it differently, but principle will be same.

Following Cassiopeia (in 60° tilt down) on Celestial sphere: If you on the equator watch 60° up to north (to compensate solar system downward angle) at vernal equinox day moment, when sun is at highest point, which is now in Pieces constellation (RA – 0h), than you would see Cassiopeia (you can check it in Stellarium when sun light is turned off). At this time/ place 23,5° Earth axis does not offset you on equator, but as you have been going up, you went up 23,5° off (hence Lacerta - 97°).

If you watch (on equator) at Autumn equinox 120° up thru ground (or went 90° north, than 30° south, than watch directly overhead)) you would again see Cassiopeia.

You may also wait to night on 60° long/ 0° lat. and wait for align galactic and azimuthal sphere (which will be in 2020 in 23. 9 at 02: 43 minutes) and you will see Cassiopeia on zenith - you can than measure galactic long., which will be 123° (+/1 1°). So you can also see that at around midnight on Spring eq. you see (logically) stars/ signs of mid-day in Autumn eq.

It may help that 60° angle is down in direction away from galaxy center: like you are on top of the roof representing solar system, behind you back is Milky way center and solar system plane is falling down (at 60°) toward Cassiopeia.

In Summer solstice at night you can clearly see that on -23,5° you are seeing Sagittarius at your zenith which is in 0° galactic longitude. In mid EU – 50° this is -23 – 50 → 73° south from zenith, so only about 20° above the ground. Ecliptic is highest (hence summer), but zodiacal signs at night are lowest.

In Winter solstice you can clearly see that galactic plane (signs in galactic plane) and ecliptic (zodiacal signs) are again crossing. You can see in night on 23,5° (to compensate Earth axis tilt) galactic sing Auriga (180° gal. long.) above your head. Ecliptic (zodiacal signs) reaches in north at night highest point – you can see top of Gemini reaches less than 20° from zenith (in day ecl. is in its lowest – hence winter).

Double check -if you compare Galactic (Polaris Galacticum Borealis) and celestial north (Polaris) you will find out, that link continues to Cassiopeia. But key is here Galactic and Ecliptic north - which goes from gal. n. to 97° (Lacerta).

Fourth picture shows galactic, ecliptic plane with celestial equator – shows that ecliptic is 60° (author claimed 63°, but corrected it - anyone can get wrong heh?))

Appendix Right Ascension define coordinates of object on Celestial sphere in analogy of longitude on Earth, but since Earth is rotating, it set 0 longitude like 0 hour at the moment of vernal equinox – when sun is at highest point on 0° lat, which is now in Pieces (Greek had it in Aries and Babylonians in Taurus – it shifted due to precession). Than the hours/ min/ sec define how long behind this point object is. To get RA working in your time/ place, you have subtract RA value from local sidereal times – it so called Hour angle. If you set value to you telescope with equatorial mount (its RA value) you will find the object.

Six hours define same way highest point of sun in summer solstice, 12 h in Fall equinox, 18 h winter solstice. This celestial day is only 23: 56 and 4 sec. long (4 min earth rotates to offset around sun rotation, In year this will add up to one day).

Declination defines position on Celestial sphere: to get you local Dec, you just subtract from Dec your latitude - means how far from your zenith object is, minus value means south direction/ plus - north.

Answer 3

I think the easiest answer is "in the same direction that the north galactic pole (NGP) is from the north celestial pole (NCP)."

Which makes it easy to see that the answer is simply the right ascension of the NGP in celestial coordinates, which was defined as 12h 49m in B1950 coordinates, was 12h 51m in J2000 coordinates, and is currently about 12h 53m (mid 2024). See https://en.wikipedia.org/wiki/Galactic_plane

Note that in mid-2024 coordinates, the maximum angle between the ecliptic plane and the galactic plane (the inclination of the galactic plane) is just over 63 degrees: 60.0046 degrees, since the current precessed position of the NGP has Declination 26.9954.

ChatGPT offers this code to precess the NGP to the date given in the code (2024-07-01). (I've tested that it properly reproduces the J2000 coordinates, and it looks good otherwise):

from astropy.coordinates import SkyCoord, FK4, FK5
from astropy.time import Time
import astropy.units as u

# Define the coordinates of the North Galactic Pole in B1950
ngp_ra_b1950 = 192.25 * u.deg
ngp_dec_b1950 = 27.4 * u.deg

# Create a SkyCoord object for the NGP in the B1950 frame
ngp_b1950 = SkyCoord(ra=ngp_ra_b1950, dec=ngp_dec_b1950, frame='fk4', equinox='B1950.0')

# Define the target date for precession
date_mid_2024 = Time('2024-07-01')

# Transform the coordinates to the target date in the FK5 (J2000.0) frame
ngp_2024 = ngp_b1950.transform_to(FK5(equinox=date_mid_2024))

# Print the precessed coordinates
print(f"RA (2024.5): {ngp_2024.ra.to_string(unit=u.hr, decimal=True)}")
print(f"RA (2024.5): {ngp_2024.ra.to_string(unit=u.hourangle, sep=':')}")
print(f"Dec (2024.5): {ngp_2024.dec.to_string(unit=u.deg, decimal=True)}")