Do white dwarfs lose mass as they fade to black dwarfs? Is there a correlation between temperature, mass, and radius?

Question

I'm having a difficult time understanding certain behaviors of white dwarfs.

I understand how mass is lost in the red giant to white dwarf transition process. I understand that white dwarfs can accumulate mass from a partner binary or some other source and I'm aware of the 1.4 M☉ Chandrasekhar limit. I get that they're not losing all their mass; in general they're just cooling and in the end there will be a lone black chunk of crystallized material floating forever in the void. I know they are really insanely hot when formed and very cold after an unfathomable amount of time. But is it that same, initial mass, or does it somehow diminish?

But is that initial mass the mass the remnant has after its cooled to a black dwarf? I guess what I'm asking is that I'm trying to figure out if there is a correlation between temperature, mass and radius. Some sort of equation?

Answer 1

The mass of a white dwarf continues to be that which it was "born" with. It will not change significantly unless it accreted material from a companion.

The radius of a white dwarf is, to first order, given by the "mass-radius relationship" and this relationship does not involve the temperature.

The mass-radius relationship is appropriate for a "cold" star. "Cold" in this context mean that the pressure that supports the white dwarf is only dependent on density, which is the case for the degenerate electrons in the interior, which have kinetic energies much greater than their thermal energy.

However, degenerate electrons also have excellent thermal conductivity, so white dwarf interiors are isothermal. Yet, they have a density gradient - denser in the middle and less dense answer move outwards. At some point close to the surface the electrons are hot enough to stop being degenerate and the gas pressure becomes temperature sensitive.

What this means is that the radius of a hot white dwarf is bigger than that of a cold white dwarf of the same mass. The effect depends on both the mass of the white dwarf (bigger for lower mass white dwarfs with lower surface gravities) and its age (since white dwarfs cool as they get older).

This effect is not negligible and needs to be properly modelled in order to understand the luminosities of white dwarfs.

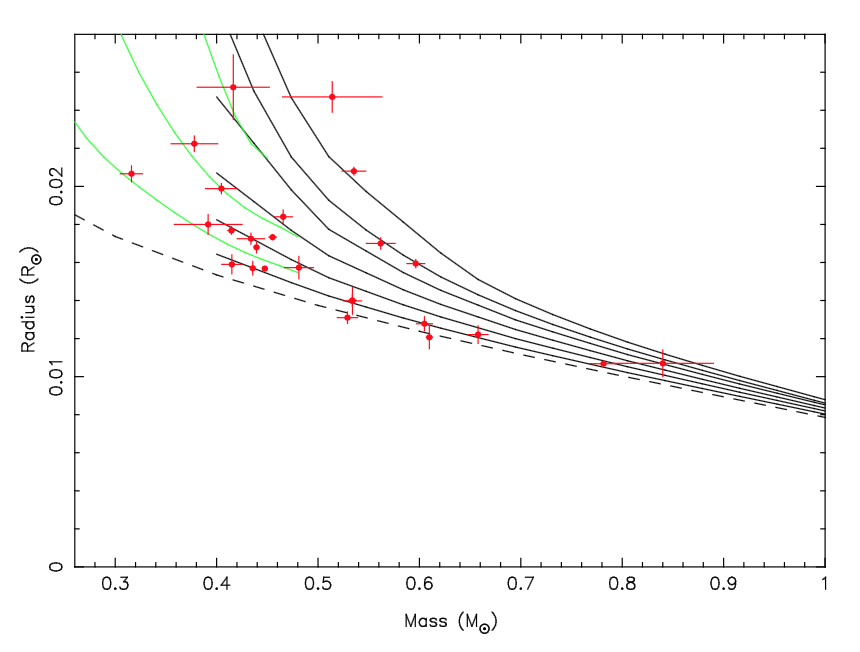
The plot below is from Parsons et al. (2017) and shows (as points) measurements of masses and radii of white dwarfs in eclipsing binary systems. The lines are model curves for white dwarfs with surface temperatures ranging from zero (dashed line) to 60,000 K (appropriate for a very young white dwarf) in steps of 10,000 K. Clearly, there is not a unique mass radius relationship and the radii do apparently depend on temperature (and core composition), as the model curves suggest.

Answer 2

The mass should be thought of as set by the history of the white dwarf, and it really doesn't change (unless there is mass transfer from a companion, but you know about that). The radius is then set by the mass, and it really doesn't change either, because the pressure comes from the internal kinetic energy which is extremely large, and is set by the quantum mechanics of the degenerate electrons. Also, the white dwarf is not really very luminous, such that it will never, in its entire lifetime, emit much energy compared to the vast amount that is already in there, and that means very little change in radius. So given the mass, there is really only one radius that is appropriate for the white dwarf, which can be found from a formula called the "mass-radius relation" for a white dwarf.

Then the last thing you asked about is the temperature. This is almost entirely separate from all the other things we've talked about. It just depends on the age of the white dwarf, as the dwarf cools, so there is no formula that connects temperature to radius or mass (at least not in an important way, there is always tiny connections because the white dwarf is not fully degenerate).