The core temperature of the Sun is approximately 15 million Kelvin. I am interested in understanding the core temperatures of main-sequence stars with various spectral types and surface temperatures, such as Proxima Centauri and Sirius. Additionally, What is the relationship between the core temperature and the surface temperature in main-sequence stars with solar metallicity? (A table or a graph showing the corresponding surface and core temperatures)
1 Answer
There is no straight nor unique relation between a star's observable surface temperature and the core temperature. Thus this cannot be answered in the general way this is asked - and this somewhat is a non-answer showing the difficulties in answering it.
The common, and readily observable property is the star's surface temperature which relates to the absolute brightness and mass via power laws, observable e.g. in the Hertzsprung-Russel diagramme: 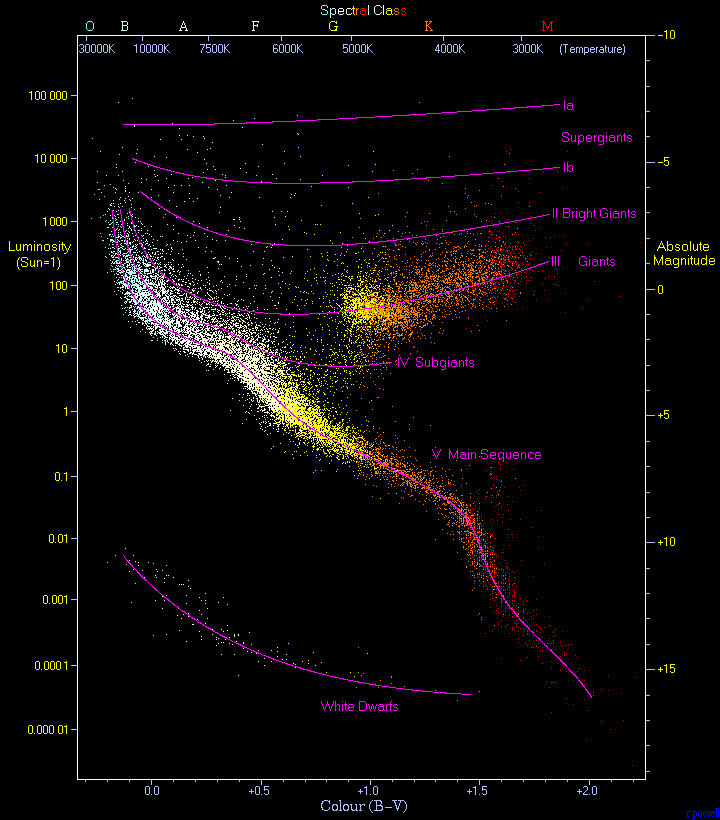
Note in the diagramme that the temperature is on the horizontal axis shown at the top (and decreasing to the right), and the (absolute) brightness on the star shown on the vertical axis. Notable, there is not an unambiguous relation between the surface temperature and the (absolute) brightness as shown on the vertical axis. As thus, there cannot be a unique relation between the surface temperature and the core temperature either as the (not shown) variables here are the radius, mass and chemical composition of the stars. E.g. the radius and mass of the stars distinguish whether a star with a given surface temperature is (still) on the main sequence or whether it is a massive giant star at the end of its life in the giant branch.
The question is further complicated the fact that main sequence stars have two competing sources of energy: the pp-cycle (for lower mass stars and lower core temperatures) and the CNO cycle for higher-mass stars with higher core temperatures. E.g. refer to this lecture summary. Thus one cannot simply extrapolate from known values, but it needs more detailed models.
The metallicity (thus chemical composition) also plays a role in the central temperature. The more heavier elements there are, the hotter the core will get.
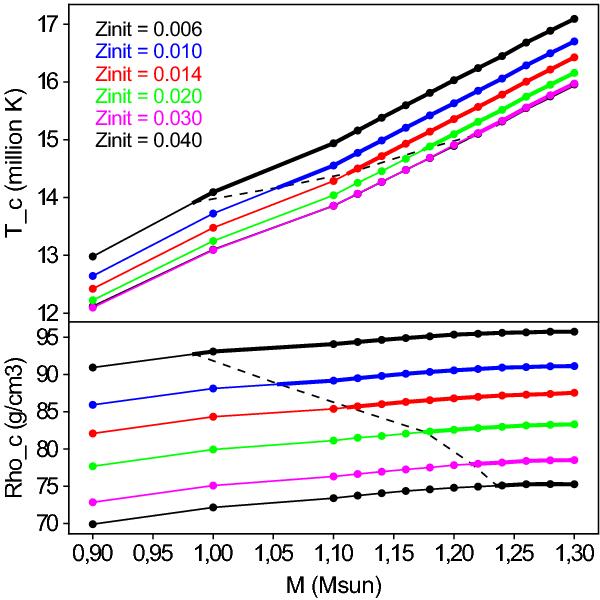
One paper I found which shows the impact of these parameters in a small mass range around one solar mass is Mowlavi et al (2012):
You find quite a nice summary in the lecture notes by Onno Pols in figure 10.1:
 There you see the tracks of stars of different masses in the HR diagramme (left) and the track of the very same stars in the core temperature-core density diagrammes with zero-age main sequence denoted by the dotted lines (the dashed in the rhs diagramme show different equation of state regions).
There you see the tracks of stars of different masses in the HR diagramme (left) and the track of the very same stars in the core temperature-core density diagrammes with zero-age main sequence denoted by the dotted lines (the dashed in the rhs diagramme show different equation of state regions).
Reading from those graphs it's roughly $T_{eff}$ to $T_c$ at the time of ZAMS:
Teff [K] : Tc [10^6K] : Mass [Msun]
5620 14.1 1
9330 21.4 2
12020 24.0 3
16980 26.9 5
20890 29.5 7
25110 31.6 10