8
$\begingroup$

Since the cosmological constant is not required to explain that the universe seem to be expanding, why do we have it?

What other factors cause us to have that constant?

Background: Without the cosmological constant, distant stars should be affected by a great redshift. The amount of redshift is a function of their distance from us. This is due to gravitational time dilation. We are looking 13 billion years into the past, where the universe was very dense. Those stars should be experiencing extreme gravity, causing Einstein shift.

Since we DO have the cosmological constant, we are now looking for other explanations for the redshift.

Improve this question
$\endgroup$
9
  • $\begingroup$ The cosmological constant is absolutely needed to explain the precise nature of the expansion of the universe (accelerating expansion). See Nobel Prize in Physics for 2011 (nobelprize.org/nobel_prizes/physics/laureates/2011/press.html). $\endgroup$
    – astromax
    Commented Jan 10, 2014 at 15:25
  • $\begingroup$ If you were travelling into a black hole at close to the speed of light, you would probably not be able to observe it coming until you were close to the event horizon. The radius of the event horizon depends on your velocity, from your perspective. If a bright object in front of you were also moving into the hole at 1/10th the speed of light, it would still have an accelerating redshift relative to you, but in fact you could crash into it before it reaching the center of the black hole. Is that plausible? If so, couldn't a collapsing universe display those effects? $\endgroup$
    – frodeborli
    Commented Jan 10, 2014 at 15:56
  • $\begingroup$ If the universe were collapsing you would see blueshifting of galaxies not redshifting. $\endgroup$
    – astromax
    Commented Jan 10, 2014 at 16:09
  • $\begingroup$ @astromax Due to Doppler, perhaps. But I believe that collapse would not behave as a cookie dough deflating. Central regions of space would experience outward pull, more than making up for the inward pull the outer shell should be experiencing. This I believe would contribute to additional redshift, because outer regions will become denser, on top of the initial red shift due to distance. Basically, I think the dynamics would make us see redshift. $\endgroup$
    – frodeborli
    Commented Jan 10, 2014 at 17:16
  • $\begingroup$ This is incorrect thinking. If the universe collapses, the coordinates of space would necessarily decrease over time. All but the closest galaxies would be blueshifted, not redshifted. $\endgroup$
    – astromax
    Commented Jan 10, 2014 at 17:41

2 Answers 2

Reset to default
10
$\begingroup$
  • Reason 1:

Let's look at the Friedmann equations without the cosmological constant.

$$ \frac{\dot{a}^2 }{a^2} = \frac{8 \pi G \rho}{3}-\frac{kc^2}{a^2}$$

The term on the LHS is just the Hubble constant squared $H^2$ which can be measured the direct measurement of recession velocity of galaxies

The density term can be said to be a combination of $\rho_{matter}+\rho_{dark- matter}$ both of which can be measured directly;$p_{matter}$ by observation of matter in our galaxy and other galaxies while $\rho_{dark- matter}$ by rotation curves of galaxies.

The curvature constant $k$ can be estimated today by the anisotropy measurements in the CMBR.

As it turns out the parameters don't fit and we need more mass-energy in the universe(almost 2-3 times of that we had estimated).

So comes along Dark energy or basically the cosmological constant. Cosmological constant or the dark energy are just two ways of looking at the equation,either as just a constant or a form of mass-energy(though we have solid reasons to believe the latter).

And this is our picture of the universe today:enter image description here

  • Reason 2:

Now historically the cosmological constant was necessary for an altogether different reason.

The second Friedmann equation without the cosmological constant looks:

$$ \frac{\ddot{a}}{a} = -\frac{4 \pi G}{3}\left(\rho+\frac{3p}{c^2}\right) $$

Now this predicts for normal type of matter,the universe must decelerate.($\ddot{a}<0$)

Now,people measured the redshift of the type-1a supernovae and found out the quite paradoxical result that the universe was being accelerated in its expansion.

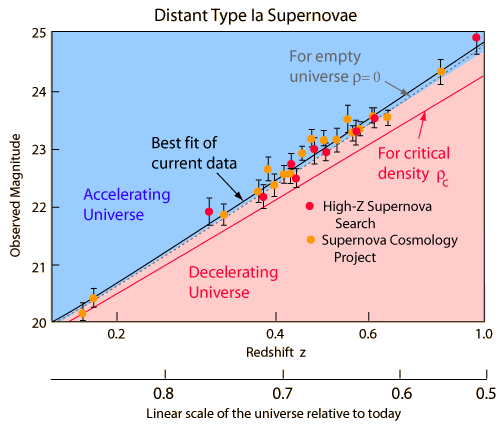
enter image description here

Since normal matter can't explain this type or behaviour,we again have to look at Dark Energy(or the cosmological constant).And so with the cosmological constant the equation becomes:

$$ \frac{\ddot{a}}{a} = -\frac{4 \pi G}{3}\left(\rho+\frac{3p}{c^2}\right) + \frac{\Lambda c^2}{3} $$

Thus $\ddot{a}>0$ is possible.

Therefore the cosmological constant is necessary to both explain the current rate of expansion and the accelerated expansion.

So finally the accelerated expansion can be explained and today we have the $ΛCDM$ model of the universe.

References:

1:http://en.wikipedia.org/wiki/Friedmann_equations

2:http://hyperphysics.phy-astr.gsu.edu/hbase/astro/univacc.html

3:http://en.wikipedia.org/wiki/Lambda-CDM_model

Improve this answer
$\endgroup$
6
  • $\begingroup$ I believe that the universe can be decelerating, or even collapse at this moment, but that collapse would in make it appear visually as if it is expanding at an accelerating rate - due to the quadratic rate of the gravitational shift. As a hollow universe in a dense shell. This is due to our own region becoming less dense relative to the most distant regions. Does those equations contradict that assertion, when removing the cosmological constant? $\endgroup$
    – frodeborli
    Commented Jan 10, 2014 at 7:21
  • $\begingroup$ Regarding the rotation curves of galaxies; how can we know the temperature of the empty space as a function of distance to us? Can we be sure that the universe is was not brighter in the past (in other words distant to us)? More photons in travel in a region should contribute to the rotation curves in that region. $\endgroup$
    – frodeborli
    Commented Jan 10, 2014 at 7:45
  • $\begingroup$ What do you mean by this "I believe that the universe can be decelerating, or even collapse at this moment, but that collapse would in make it appear visually as if it is expanding at an accelerating rate - due to the quadratic rate of the gravitational shift?" $\endgroup$
    – Sandesh Kalantre
    Commented Jan 10, 2014 at 7:59
  • $\begingroup$ The rotation curves are obtained either using the velocities of actual stars or the lines in atomic hydrogen,basically the 21cm line.There are other ways of detecting dark matter,for example dark matter can also cause gravitational lensing.E.g.:en.wikipedia.org/wiki/Bullet_Cluster $\endgroup$
    – Sandesh Kalantre
    Commented Jan 10, 2014 at 8:03
  • $\begingroup$ Since the universe appears to be expanding, it should mean that distant stars affect us with gravity less as a function of time. Distant stars, on the other hand should appear to be more affected by gravity as a function of time (due to distance). I then assume time dilation shift. If, on top of that effect, the universe is collapsing - and gravity propagates at c - distant regions should be more affected by gravity than close, experiencing a similar effect to the sound barrier - but for gravity. The sum of this should amount what we call the cosmological constant, I believe. $\endgroup$
    – frodeborli
    Commented Jan 10, 2014 at 9:46
-2
$\begingroup$

I propose the following model:

The universe consists already of a giant central black hole attracting our milky way and all other galaxies. The observed red shift can be explained by the 1/r² law of gravitation: galaxies that are nearer than our one to the central black hole have higher speeds towards it than we have. So we see them moving away from us. Galaxies that are further away from the central black hole than our one don't move so fast towards it as we do. So looking at them we see them escaping from us as well. The black hole and our distance to it are so big, that the field gradient is quite low, so we experience no tidal forces. Adjusting Newton's 1/r² law by Einstein's general relativity field equations does not make a big difference to this model. The general pattern of galaxy motion relative to each other remains the same.

How can these claims be verified? Here is the principle algorithm. It can be implemented and executed even on a PC:

Take all recorded quasar spectra from the known quasar catalogs. Normalize their spectra to zero red shift. Compare each spectral signature which each other. Try reasonable compensation for differences according to dust or other noise adding effects. If you find two identical or similar ones, check the respective quasar's positions. If their angular positions differ by several degrees, you have some evidence. Because you found the same quasar twice: Once seen in direct (curved) line, and once its light wrapped around by the central black hole's gravitational field. Twin quasars are already known but turned out to be the result of "small" (means: a massive galaxy in our line of sight to them) gravitational lens effects. In these cases their seeming positions differed by some arc seconds or minutes. A number of twin quasars with significant (several degrees) angular position differences or even at opposite sites of the universe would be a proof for the above model

Improve this answer
$\endgroup$
8
  • $\begingroup$ I've been through the a similar line of thought as you, and I also believe that due to 1/r^2 you would see uniform redshift. While falling toward the hole, you would see an accelerating red shift in every direction. I've taken the line of thought a little further: once you cross the event horizon, tidal forces will rip everything apart into the the primary elements - electrons, neutrons or whatever. When this mixture comes closer to the absolute center - they will be less affected by tidal forces and can start to form hydrogen, stars and new black holes - inside the first black hole. $\endgroup$
    – frodeborli
    Commented May 14, 2014 at 9:43
  • $\begingroup$ The problem with finding two identical quasars is that you'll be looking at it vastly different distances - a young quasar and an older quasar. You could also imagine that if the milky way was falling toward a giant black hole, you'd see ourselves directly "behind" us. The mirrored milky way would appear to be on collision course with us. The idea came as an alternative explanation for the "Great Attractor" and the fact that the Andromeda Galaxy appears to be on collision course with us, much faster than the galaxies mass can account for. $\endgroup$
    – frodeborli
    Commented May 14, 2014 at 9:51
  • $\begingroup$ I've asked about this in the past as well at marilynvossavant.com/forum/viewtopic.php?t=376 $\endgroup$
    – frodeborli
    Commented May 14, 2014 at 9:58
  • 2
    $\begingroup$ -1 There could not be a central black hole because the universe has no center. See this question: astronomy.stackexchange.com/questions/669/… $\endgroup$
    – called2voyage ♦
    Commented May 14, 2014 at 12:31
  • $\begingroup$ @called2voyage How can you be completely certain that there is no center? Are there any certain ways to know that the universe is NOT inside another black hole? That all matter we see was ripped apart when crossing the event horizon of a black hole in an "earlier" universe, and now have been forming new stars after billions of years inside this superhole? Just curious as to how anybody can be certain and just shoot down that idea. Because a black hole certainly has a center when viewed from outside. $\endgroup$
    – frodeborli
    Commented May 14, 2014 at 13:14

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .