What is the difference between albedo, absolute magnitude or apparent magnitude? I can’t really tell what’s the difference between them.
-
6$\begingroup$ Hi Angela, welcome to AstronomySE. Have you looked up the three terms on Wikipedia? You'll get a simple explanation of each term there. If you still have a question that Wikipedia doesn't answer, please use the search field to see if it's already been asked here, and if not, we would welcome your question :-) $\endgroup$– Chappo Hasn't ForgottenCommented Feb 3, 2019 at 5:59
-
$\begingroup$ Albedo is a property of the given body, absolute magnitude depends also on illuminating body and apparent magnitude on distance from observer in addition. $\endgroup$– Leos OndraCommented Nov 16, 2023 at 19:12
2 Answers
Albedo is a measurement of reflected light. It varies between 0 and 1, with 0 being completely black (reflecting no light) and 1 being completely white (reflecting all light). The albedo of coal is about 0.04, which is actually very similar to the albedo of our moon. The Albedo of ice is about 0.7. Only planets and other small objects are described using Albedo because they cannot make their own light, and so its apparent brightness is due to reflecting sunlight.
Absolute magnitude is a measure of how much light a star is emitting overall. Absolute magnitude is related to a bunch of other properties of the star, mainly how massive it is and at what stage of its lifetime it's in.
Apparent magnitude can be used to describe both stars and planets. It is simply a measure of how bright the object appears to us. An illustrative example is Sirius and Canopus. Sirius is the brightest star in the sky, but it is bright because it is close, only being 8 light years away. Canopus is the second brightest star in the sky, and appears about half as bright as Sirius, but is about 300 light years away, which means the star itself must much brighter than Sirius. If we check the absolute magnitudes and do some maths (Sirius absolute magnitude = +1.42, Canopus = -5.71, the more negative the number the brighter the star, brightness difference = 2.5^(5.71+1.42)) we get a brightness difference of 680 times between Canopus and Sirius.
-
$\begingroup$ IIRC, apparent magnitude also depends on the color -- stars which emit in wavelengths where our eyeball is less sensitive have smaller apparent magnitude. // Unless I'm confusing my terminology. $\endgroup$ Commented Feb 5, 2019 at 15:02
Here is how I understand these terms.
- Apparent magnitude is what you can see, or measure from where you really are and where the object really is.
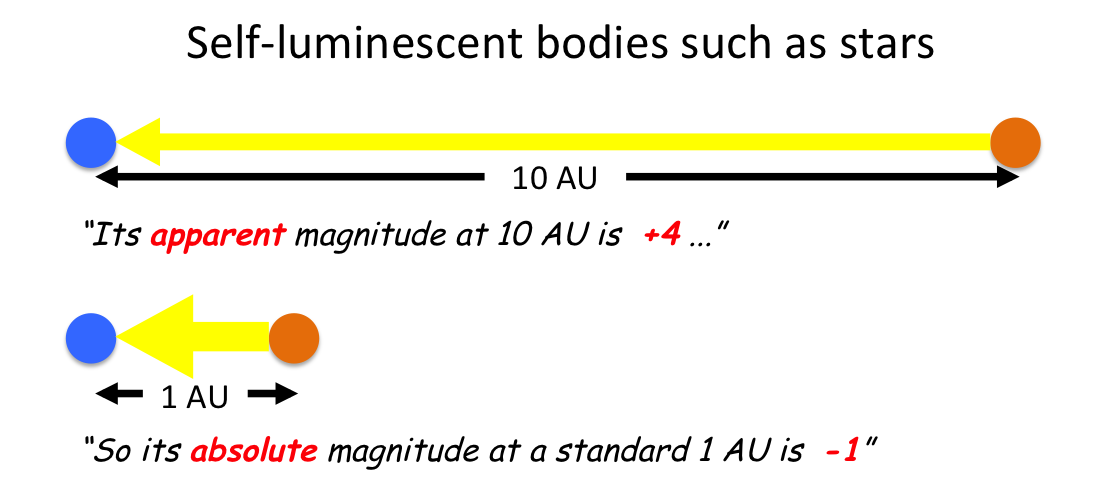
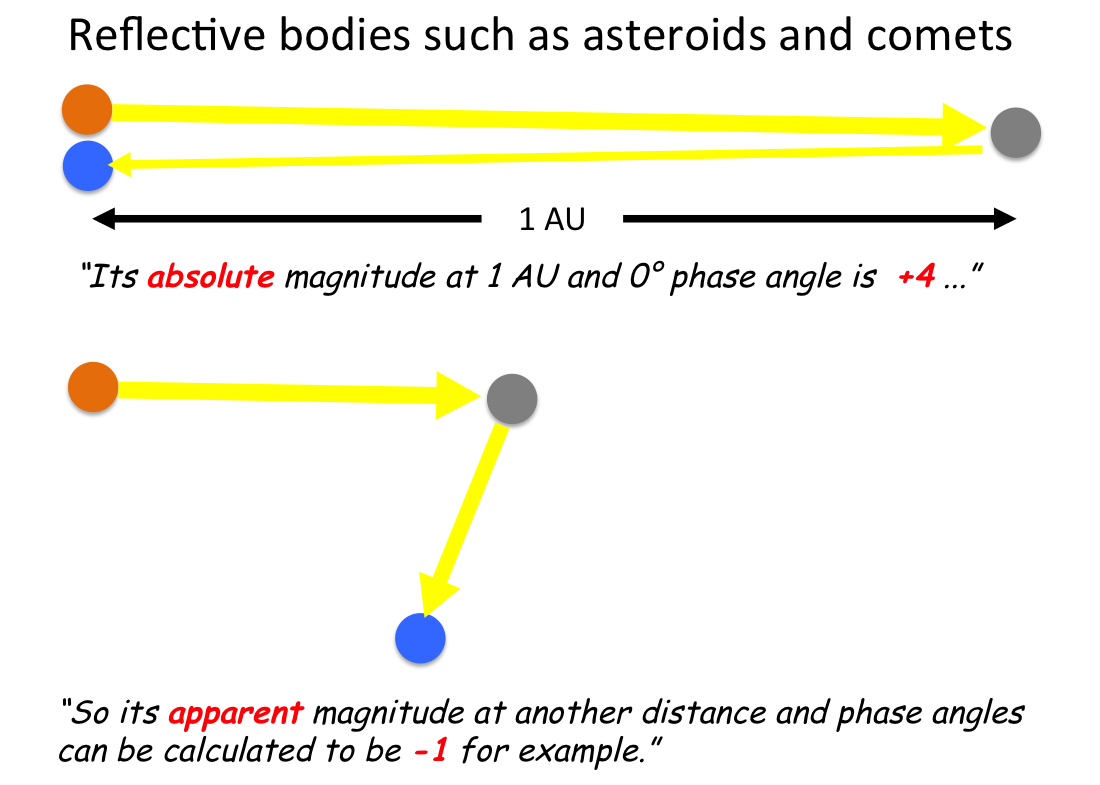
- Absolute magnitude is a mathematical prediction based on a standardized distance and configuration (see below) so that you don't have to specify the particular conditions. Venus always has roughly the same absolute magnitude because it's always roughly the same size and albedo (see next), but varies greatly in apparent magnitude be cause of changes in distance and phase angle (sun-venus-earth angle).
- Albedo is a little tricky because different objects have different surface roughness and angular scattering properties. If it was a smooth ping-pong ball and had a well defined diffusivity profile, then it's easy to imagine a white, gray, or black ping-pong ball representing transitions from 1 to 0.
I've just asked Is albedo ever greater than unity? How is it calculated for various surface diffusivities and specular reflection? to get at some of those subtleties.
For stars and other objects that make their own light:
For planets, asteroids, comets, satellites (artificial and natural) and even electric cars 1, 2 that are seen in reflected light from the Sun:
-
$\begingroup$ I'm creating a youtube video on archeoastronomy and love the Absolute vs Apparent Brightness image. Do you know the creator of this image? I would love to use it if that's at all possible. Thank you! $\endgroup$ Commented Nov 16, 2023 at 18:56
-
$\begingroup$ @BrianRoss I drew this sketch myself (comic sans and all) in an old version of PowerPoint (note the shadows behind the circles) for the purpose of this post. You or anyone else are free to use it per the SE license (CC BY-SA 4.0) i.sstatic.net/uHDl4.png If you have access to PowerPoint or some more modern sketching app you could probably create a nicer looking variant in only a few minutes, at which point I think it's so simple that it would not need a citation at all. Anyway, have fun! $\endgroup$– uhohCommented Nov 16, 2023 at 21:46