I didn't find anywhere talking about this kind of eclipse seasons, except at Oxford Reference and University of Nevada, where they say that it would be 24 days. But it probably has a range of several days shorter or longer, just as regular eclipse seasons range from 31 to 37 days (Wikipedia). So what is the range of this kind of eclipse seasons?
-
1$\begingroup$ In the University of Nevada link, near the half of paragraph 4, it says “Mo-128,“ which is a reference you can click: It quotes Motz and Duveen, Essentials of Astronomy, 1977, p. 128. I found this book online archive.org/details/essentialsofastr00motz/page/127/mode/1up You can see there a diagram and (continuing on the next page) an explanation as to why it’s 24 days—no more, no less. $\endgroup$– Pierre PaquetteCommented Dec 8, 2023 at 0:24
-
$\begingroup$ Sounds good, but I can't access the link (the problem is on my side, but I can't fix it). So if you can tell me some of its content it would be great. $\endgroup$– George LeeCommented Dec 8, 2023 at 14:26
1 Answer
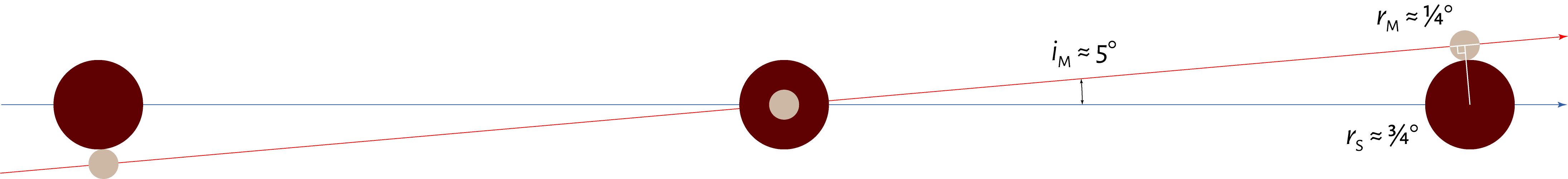
Here’s an adaptation of the relevant image in Essentials of Astronomy (my image is to scale and shows the ascending node, whereas the image in the book is not to scale and shows the descending node).
The Earth’s shadow is the dark disk; the lighter disk represents the Moon. On the right, you can see the sizes and angles involved: The Moon’s path (red line) is tilted about 5° relative to the ecliptic (blue line); the Moon’s radius is approximately 0.25°, and the radius of the Earth’s shadow is about 0.75°. It barely shows at the thumbnail size (click on the image to see it much bigger), but the white line between the centre of the Earth’s shadow and the centre of the Moon is slightly tilted (about 5° as well). This means the total length of this line is about 1°.
A little spherical trigonometry will give you the length of the ecliptic between the centre part of the image (total central eclipse) and the right part. Since the angles are small, we may use plane trigonometry without being too much in error. We thus get:
$ \displaystyle \begin{align} \tan i_M & = \frac {(r_M + r_S)}{x} \\ x & = \frac {1°}{\tan 5°} \\ x & ≈ \frac {1°}{0.0875} \\ x & ≈ 11.43° \end{align} $
The authors could have rounded the result to 12°, as they give the same measurements of 5°, 0.25°, and 0.75° for the various elements.
Doubling this gives 22.86° or, “rounded,” 24°. QED.
-
1$\begingroup$ It rounds up to ~24 days because the Earth's mean angular speed (relative to the node) is a little under 1°/day. $\endgroup$– PM 2RingCommented Dec 9, 2023 at 4:15
-
$\begingroup$ This explanation doesn't state that it's "24 days – no more, no less". It just gives the average duration. $\endgroup$ Commented Dec 10, 2023 at 21:34
-
$\begingroup$ The fact that the white line is tilted (~2.5°, not ~5°), doesn't affect it's length, since we deal with circles. The total length is ~1° simply because 0.75+0.25=1. I guess it's just that two sentences were accidentally swapped. $\endgroup$ Commented Dec 10, 2023 at 21:35
-
1$\begingroup$ or you can just say: tan 85°=11.43. But really we need to make: 1. tan 87.5° (or more exactly 87.55), because the white line isn't perpendicular to either the blue or the red line, but it's just in between. 2. The average radius of Earth's shadow is (Earth angular diameter from the Moon - Sun's diameter) = 1.9° - 0.53° = 1.37°. So 1.37/2 + 0.52(=Moon's diameter)/2 = 0.945. So tan(87.55) * (0.945/2) = 11.04°. Which would make a season of (11.04*2/0.986) = 22.39 days. $\endgroup$ Commented Dec 10, 2023 at 21:36
-
$\begingroup$ Actually, the white line is perpendicular to the red line, at least in the original drawing. $\endgroup$ Commented Dec 10, 2023 at 21:36