I assume that the A ring is the densest, but I might be wrong. Nevertheless, I failed to find any explicit information over the net about the minimum and maximum of densities for the different rings. As a less practical question: what would one see upon entering one of the denser rings? Would it be obvious that it is a dense but thin (few metres - 1 km) cloud of particles, continuously bombarding the spacecraft irregardless whether it orbits along within the ring, or one would not see more than just a distant haze (if lit by the Sun) that spans over the horizon?
$\begingroup$
$\endgroup$
6
-
$\begingroup$ @RoryAlsop Would a 'what would this look like' include images, or something else? $\endgroup$– HDE 226868 ♦Commented Nov 4, 2014 at 16:05
-
$\begingroup$ I was thinking images or a description, and to be honest, MBR's update looks like it could fit the bill, so unless a better answer comes along, he'll probably get the bonus. For some reason I wasn't expecting it to look like fog... $\endgroup$– Rory AlsopCommented Nov 4, 2014 at 16:19
-
$\begingroup$ @RoryAlsop Hadn't seen MBR's edit. Yep, that should be good. $\endgroup$– HDE 226868 ♦Commented Nov 4, 2014 at 19:14
-
$\begingroup$ Thanks @Rory for raising the visibility of my Q! I'm not familiar with the bounty system, is it awarded when I accept an answer (and that answerer is awarded the bounty), or the bounty is independent of my decision? $\endgroup$– István ZacharCommented Nov 6, 2014 at 14:59
-
$\begingroup$ holy crap - Saturn's rings are only a few meters thick?!?. WTF $\endgroup$– FattieCommented Jul 11, 2016 at 14:30
|
Show 1 more comment
2 Answers
$\begingroup$
$\endgroup$
2
Elements of answer:
It is not an easy question, as we lack of data to constrain strongly the density and mass of Saturn's rings.
However, a first clue of their density is their optical depth (that is a measure of the transparency of a medium). The densest rings are the one of the main ring system (rings A, Cassini Division, B and C) plus ring F; they are characterized by optical depth larger than 0.1 (up to about 5 for the densest part of ring B). Rings in the faint ring system (E and G) have very small optical depth (lower than $10^{-5}$).
With this regard, B is indeed the densest ring of Saturn's rings. (edit) Looking around ([1] and [2]) you can find numbers that help to figure out the density of this ring. Knowing its mass (about $2.8\times10^19$ kg) and its extend (from 1.527 to 1.951 Saturn radii, with a typical thickness of 100 m), you can deduce a mean density of about 10 kg.m$^{-3}$ (about 10 times denser than the terrestrial atmosphere at sea level).
Just for fun:
This gives you an idea of what means the optical depth. In the densest parts of ring B, optical depth is between 2.5 and 5, so you'll definitely notice you're in the ring. It is probably more complicated than that (with ring particles ranging from dust size to house size), but you get the idea.
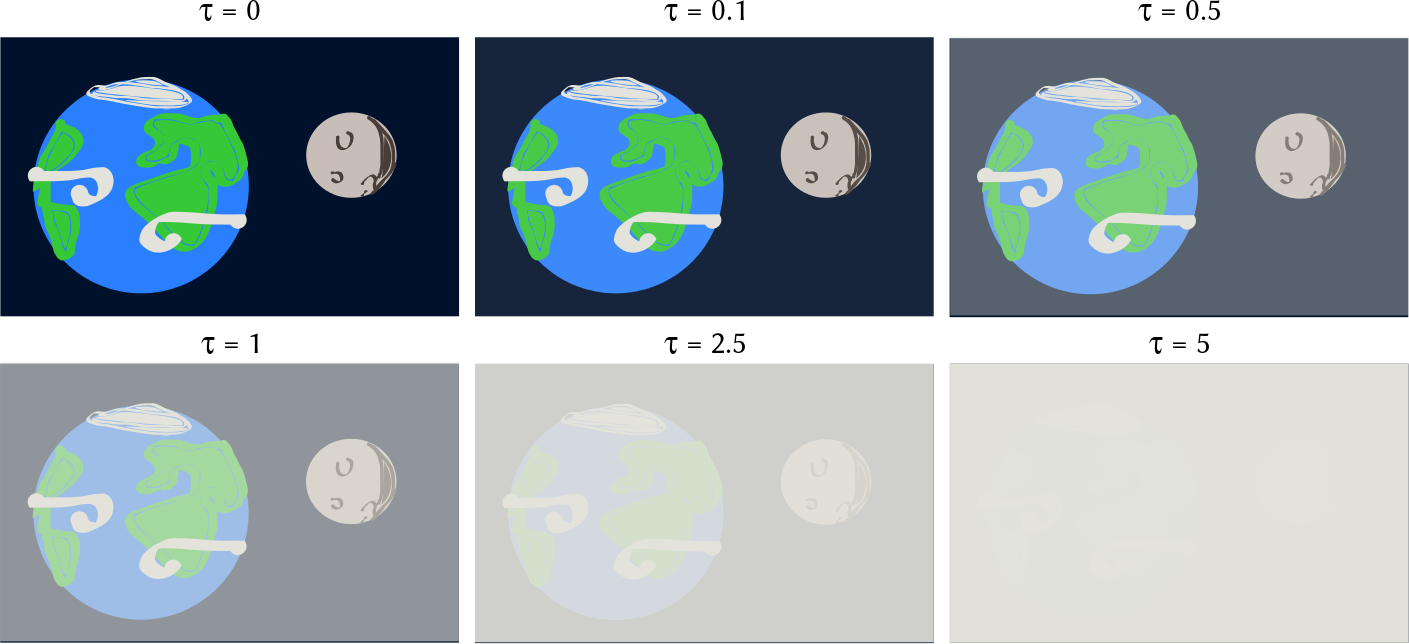
Side note:
Optical depth is a measure of transparency of one medium; it measures how radiation is neither scattered nor absorbed by the medium. It expression is given by
$$\frac{I}{I_0} = {\rm e}^{-\tau}$$
where $I_0$ is the source intensity, $I$ the observed intensity, and $\tau$ the optical depth.
Sources:
-
$\begingroup$ Since your answer is still above DilithiumMatrix's: I think you are off by many orders of magnitude. The typical mass of the rings of Saturn is 10^19 kg, not 10^9 kg. For rings 10^7 km wide and 100m thick, this gives a density of 10 kg/m^3, 10 times air and 1/100th of water. As the rings are mostly ice, this means 1% by volume. There's at least an order of magnitude uncertainty there, but the dense parts of the rings are way denser than the value you give (0.00167 g/m^3). $\endgroup$ Commented Jan 27, 2023 at 23:20
-
1
$\begingroup$
$\endgroup$
The denser rings are actually very comparable in mass density to that of air. Because of their larger particle/particulate sizes, the number density is much much smaller.
e.g.
Ring A: Radius ~ 137,000km, thickness ~ 10m, surface density ~ 20 g/cm^2
so a density of about 0.02 g/cm^3, which is about 10 times more than air, and 100 times less than water.
Much, much more info here:
http://nssdc.gsfc.nasa.gov/planetary/factsheet/satringfact.html
