I'm wondering what the farthest object we know of, as measured only by parallax methods, from either earth or satellite based telescopes, and at either visible light or radio wavelengths.
Basically, if we had no other way of measuring distances, what would be the farthest object we knew about? I'm guessing this measurement would probably be one from Gaia, it being the latest telescope to measure such things TTBOMK.
The first data release from GAIA was in 2016 so fairly new. If you want to be certain you should take a look at it because as you say, GAIA will no doubt be the source of the most accurate parallax measurements in the near future. Speaking of accuracy, I believe that this is the essence of your question because it basically depends on how uncertain measurements you are willing to accept. Basically, modern values of parallaxes are very small numbers, e.g. 0.1 milliarcsec which means a distance of
d(pc) = 1/(parallax in arc-seconds)
around 10,000 pc (one parsec is 3.26 light years). But the important point is the accuracy of these estimates. Clearly if your measurements is 0.1 milliarcsec, but the accuracy is 1 milliarcsec, it would be going out on a limb to postulate that you have measured a distance of 10,000 pc. So the real answer to your question is another question "to what accuracy?" So if you want something like 10 or 20 % accuracy you are more or less limited to our neighbourhood, say some 200 pc. If you check out ESA's website on stellar parallax, they will go up to 500 pc but it really depends on what level of accuracy your work requires.
If you check out the GAIA satellite then the aim is actually to go down to an accuracy of 24 micro-arc seconds so whatever object is measured to be farthest (with a given accuracy) is sure to replaced as the data from GAIA is analysed.
For fun, you might wanna look at this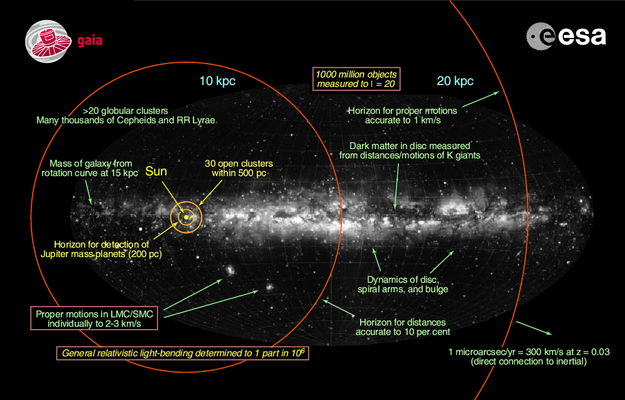 
also found at the GAIA website.. I think it provides a nice visual guideline to what distances we can expect (and it also nicely shows a ring at 500 pc - the limit of fairly accurate measurements from Hipparchos!)
It depends what parallax uncertainty you are prepared to tolerate. Very Long Baseline Interferometry (VLBI) at long wavelengths currently provides the most precise parallaxes. Parallaxes to bright radio sources measured in this way can have precisions of around 10 microarcseconds (see for example Reid et al. (2014).
According to the review by Reid & Honma (2014), the most distant source with a VLBI-based trigonometric parallax is the star forming region W49N. The source has a parallax of $90\pm 6$ microarcseconds and a corresponding distance of $11.1 \pm 0.8$ kpc (Zhang et al. 2013).
The precision of these parallaxes is similar to what is likely to be possible with Gaia for the brightest stars (e.g 5-16 microarcseconds according to https://www.cosmos.esa.int/web/gaia/science-performance ). Only the most luminous giants will be this bright at distances of $>10$ kpc.
The most distant parallax measurements are made with very long baseline radio interferometry, mapping the apparent motions of clumps of water vapor masers. I think the distance record is still held1 by NGC 4258, but the method is applied to many of the galaxies in the Local Group, as well as to a number of Galactic water masers at distances beyond 10 kpc.
[1] See a recent 2013 paper giving ~7.6 Mpc or 25 million light years away.
