When we see a crescent Moon that means it should be seen a half Moon from another position in the sky, because the terminator line (i.e. the line that separates the bright and dark areas of the Moon) is in fact a meridian. And a Meridian is a straight line which is seen curved when it's looked from an angle.
So when we see a regular narrow crescent Moon on the Earth, how far should we be from the planet in its orbit to be in front of that Meridian?
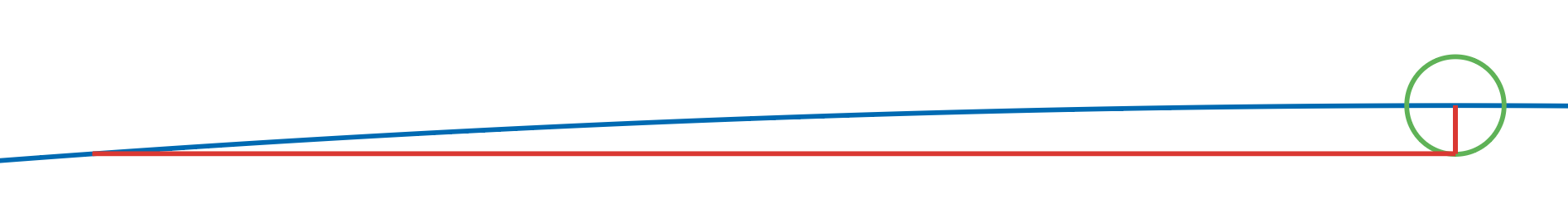
In the image below, the blue curve is the Earth’s orbit, and the green circle is the Moon’s orbit around the Earth. The corner of the red angle is the position of the New Moon. A crescent would be slightly to the left or to the right of this point, but it doesn’t change much if we restrict ourselves to a “narrow crescent” as you mention in your question.
In order to see the Moon half-lit (as a “quarter”), while staying along the Earth’s orbit, we would need to be at the leftmost point of the red angle, at the far left of the drawing.
Given that the Moon’s orbit is roughly 384,400 km in radius, a rough measurement on the drawing gives the distance to the intersection of the blue curve (Earth’s orbit) and red line (line of sight for half-moon) as approximately 5.3–5.4 million kilometres EDIT: I had forgotten to multiply by 2 (as my circle for the Moon’s orbit was 384,400 in DIAMETER), so the answer is ≈10 million kilometres.
Your actual mileage may vary. Slightly.
