Why are IAU constellation boundaries not rectilinear?

Question

According to https://www.iau.org/public/themes/constellations/

Eugène Delporte of the Royal Observatory in Brussels presented proposals for a clearly defined system of constellation boundaries drawn along lines of right ascension and declination

yet the text files defining the constellations' boundaries such as https://www.iau.org/static/public/constellations/txt/umi.txt

13 03 16.9470| 76.3289108|UMI 
13 04 23.3937| 69.3293610|UMI 
14 02 36.1947| 69.3991165|UMI 
14 03 16.9333| 65.3996506|UMI 
15 41 19.0957| 65.6023483|UMI 
15 40 12.1512| 69.6009445|UMI 
16 31 21.8550| 69.7383041|UMI 
16 28 52.9698| 74.7347870|UMI 
17 26 08.7929| 74.9033127|UMI 
17 20 52.2971| 79.8953476|UMI 
17 50 37.4449| 79.9857483|UMI 
17 26 53.3353| 85.9495697|UMI 
20 34 53.0328| 86.4656219|UMI 
20 33 19.5253| 86.6306305|UMI 
22 54 02.5599| 86.8368912|UMI 
22 37 02.6371| 88.6638870|UMI 
09 03 19.7931| 87.5689163|UMI 
08 41 36.6601| 86.0975418|UMI 
14 12 05.5098| 85.9308090|UMI 
14 27 07.8855| 79.4449844|UMI 
13 35 14.2055| 79.3629303|UMI 
13 36 37.6845| 76.3638153|UMI 

do not go along lines of right ascension and declination (in the above, only one coordinate should change in each line).

In the GIF from that page, some line segments are clearly not radial:

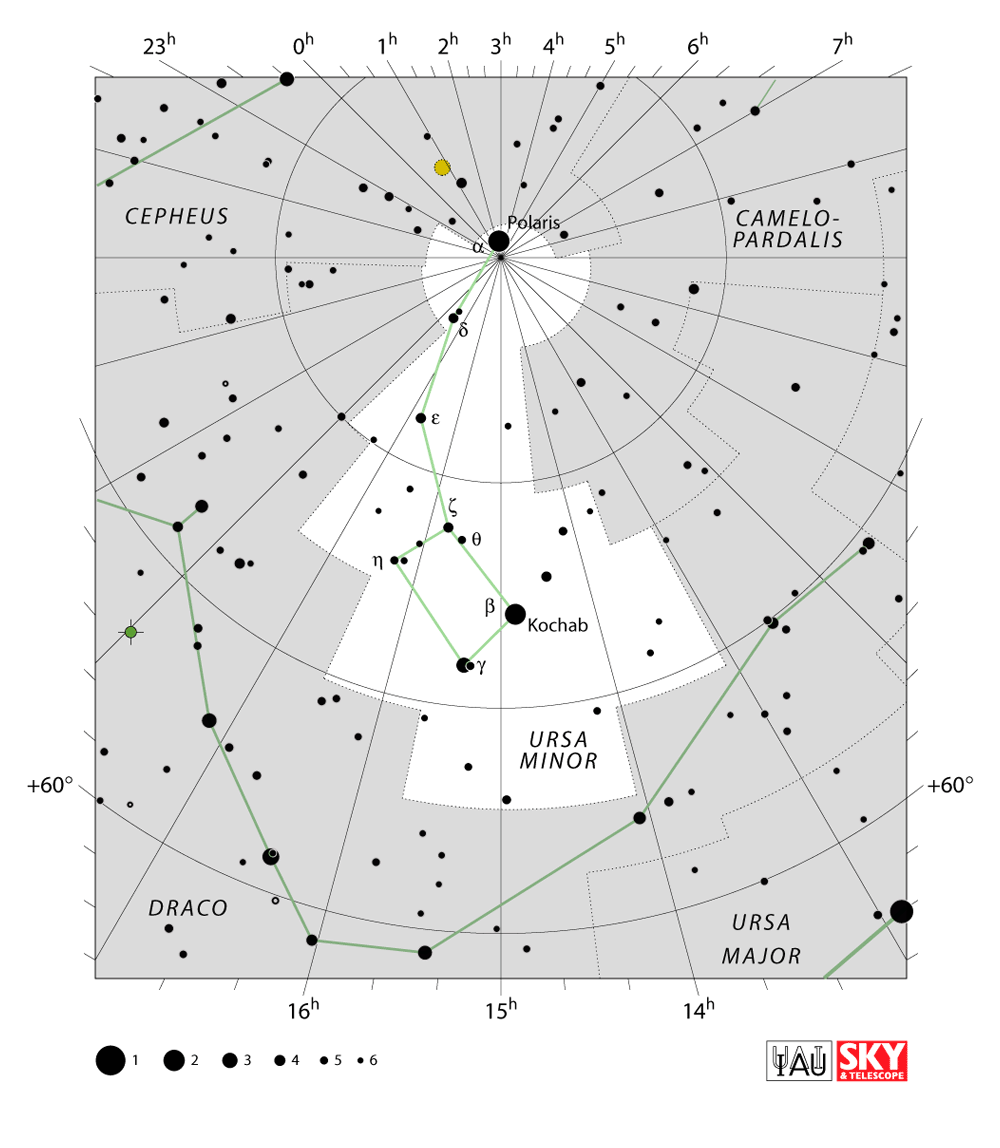
Why is this so?

Answer 1

They are... in a different coordinate system.

The boundaries were following straight lines in RA and DEC in the coordinate system for the epoch of B1875 which was used for convenience reason when the boundaries were defined in 1928 by Eugène Joseph Delporte.

The boundaries developed by Delporte used data that originated back to epoch B1875.0, which was when Benjamin A. Gould first made his proposal to designate boundaries for the celestial sphere, a suggestion on which Delporte based his work.

The image you show uses (very likely) the currently most widely-used epoch of J2000. The ecliptical coordinate system which defines right ascension and declination is based on the Earth axis and its orientation. And this orientation of the Earth axis in space does ever so slowly change due to precession, nutation and some other effects, thus mostly due to the torque of other bodies onto Earth.

Thus for comparisons purposes astronomy introduced the so-called epoch, which gives the coordinate system for a specific point in time, the epoch. Any results from observations are usually coordinate-transformed and communicated in the coordinates of the epoch (RA and DEC with respect to the mean equinox of an epoch) instead of the coordinates as they have been actually observed (RA and DEC with respect to the true equinox). With the quite well-known rotation of Earth and how it changes, the true coordinates (RA/DEC with respect to the current time / true equinox) are readily calculated and can be used to find any object thus described; differences are relatively small (usually of the order of arc minutes), when the time difference is a few years, thus any such correction can be omitted when no great positional accuracy is required and it suffices to have the object in the field-of-view of a small to moderate-sized telescope.

There is a rotation-free coordinate system, the International celestial reference frame (ICRS), which has its origin in the bary-centre of the solar system and its axes defined by far-distant quasars which are so far that any secular motion will remain unresolvable for any forseeable future. But this is not as convenient to use as RA/DEC when you are on Earth and want to look at the night sky.