0
$\begingroup$

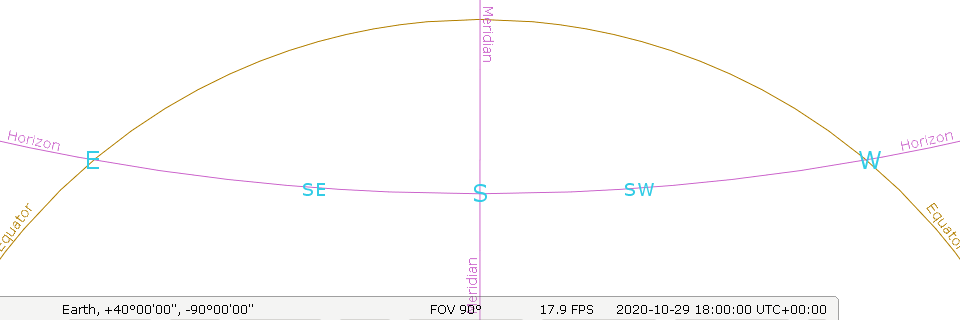
Altitude of a star at culmination = co-latitude + declination. I understand that the co-latitude is equal to the altitude of the celestial equator (since it is 90 degrees away from the NCP). I also understand that declination is the height of a star above the celestial equator.

But I am just unsure why you can directly link altitude and declination at the exact point of culmination? And why is a star reaching its highest point as it crosses the meridian?

thanks for any help.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Unless the observer is at a geographic pole, the celestial equator is not parallel to the horizon but crosses it at an angle equal to the observer's colatitude. A star's path across the sky is parallel to the celestial equator, with maximum altitude midway between the eastern and western horizons, i.e. on the meridian.

Horizon and celestial equator from 40N
Rendered by Stellarium

Improve this answer
$\endgroup$
2
  • $\begingroup$ ah, thank you. So you can say that the celestial equator is at its maximum altitude as it crosses the meridian, and this maximum altitude is equal to the co-latitude? $\endgroup$
    – Matthew H
    Commented Oct 30, 2020 at 13:12
  • $\begingroup$ @Matthew That's right. $\endgroup$
    – Mike G
    Commented Oct 30, 2020 at 13:30

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .