3
$\begingroup$

A comment under this answer states that the apparent angular speed of the Sun is 8% slower during the solstices. This is rather counter-intuitive, since the rotation speed of the Earth is constant (or close enough for the timescales considered).

Why does the Sun appear to move slower in the sky at the solstices?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

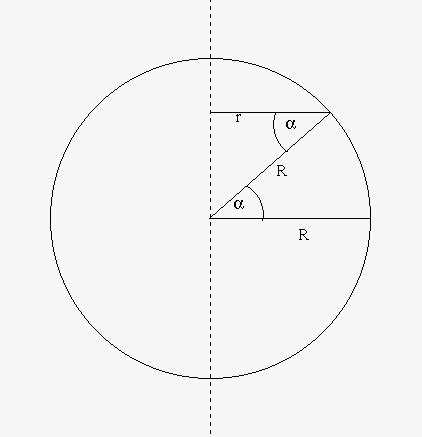
All points on the celestial sphere execute a full circle every (sidereal) day, but the speed of a point with nonzero declination is slower than a point on the celestial equator because it's traveling on a small circle, not a great circle. This is exactly the same as how a point on the terrestrial equator travels at a higher speed than a point not on the equator. Eg, a point with a latitude or declination of 60° travels at half the speed of a point on the equator, because cos(60°) = 0.5

At the solstices, the Sun is on either the tropic of Cancer or Capricorn, so it has its maximum or minimum declination, approximately ±23.4°. So its speed is cos(23.4) $\approx$ 0.9178 relative to a point on the celestial equator, or about 8% slower, as Mike G mentioned in that comment.

Here's a diagram from an answer about latitude speed by SF. on the Space Exploration Stack Exchange:

latitude speed diagram

Improve this answer
$\endgroup$
9
  • $\begingroup$ I guess I didn't phrase my question right. I wanted to know about the angular speed, because that's what is mentioned in the linked answer. $\endgroup$
    – usernumber
    Commented Mar 2, 2020 at 12:47
  • 2
    $\begingroup$ @usernumber Well, "angular speed" can be a bit ambiguous. ;) But my answer is talking about the same thing that Mike G is. Eg, in 1 hour a star on the celestial equator traces out an arc across the sky of 15°, but a star at 60° declination traces out an arc of half that length, and a star close to the celestial pole only moves along a very small arc, although its arc will have a lot more curvature. $\endgroup$
    – PM 2Ring
    Commented Mar 2, 2020 at 13:05
  • $\begingroup$ astronomy.stackexchange.com/questions/1116/… may or may not be helpful $\endgroup$
    – user21
    Commented Mar 2, 2020 at 15:58
  • 1
    $\begingroup$ Maybe it's helpful to consider the equivalent terrestrial situation. A nautical mile is defined as the length of an arc of 1 minute on any meridian of an ideal spherical Earth, so 60 NM (nautical miles) equals 1 degree on the meridian. If you travel exactly north or south along a meridian by 60 NM your latitude changes by 1°. Like the meridians, the equator is a great circle, so if you go 60 NM along the equator your longitude changes by 1°. But if you travel along the small circle of latitude 60° for 60 NM your longitude changes by 2°. $\endgroup$
    – PM 2Ring
    Commented Mar 6, 2020 at 14:53
  • 1
    $\begingroup$ +1 Useful. @usernumber, the last paragraph of this answer may or may not help. $\endgroup$
    – Mike G
    Commented Mar 7, 2020 at 2:33

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .